How to draw lines of best fit?

In many questions in Physics or often in a practical students are often required to draw a line of best fit.

So what is the line of best fit. The line of best fit is a line that is drawn through a series of points in a graph in order to determine the trend in the points. The line can then be used to determine the gradient and the y-intercept of the line if it is a straight line.

The following are tips that you can use in order to obtain the best line of best fit from a few points. You should read all of them and choose which tips is appropriate.

Tip 1

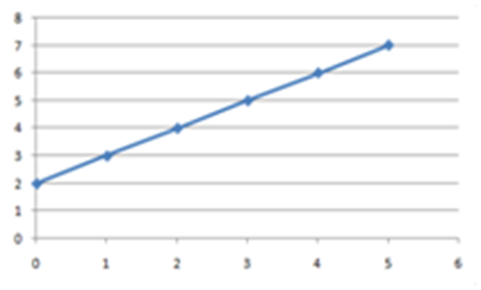
Sometimes you will obtains points that are as shown below in fig 1. Although I must say that such occurrence is quite rare. In fig 1 below you can see that the points forms an almost perfect straight line. In such a case it would be enough to choose two points far away from each other and then draw a line from one to the the other. Make sure that the line extends on both side to cover all the points.

Fig 1

Tip 2

Most of the time you will be faced with points as in fig 2 below. As such you will have to draw a line such that the points are on both sides of the lines as such. A you see from one side of the line to the other the points alternates up and down. Also make sure that two points that are closest to each other and are on different sides of the line are the same distance from the line.

Fig 2

Tip 3

In case you are unable to make the points alternate on both sides of the line from one end of the line to the other as in fig 3 below. It is acceptable to have them two by two on the same side of the line.

Fig 3

Tip 4

It may happen as in fig 4 below that one point is so far from the others that it would make no sense to include it when drawing the line of best fit. This point is called an outlier and it is often the result of an error in calculation, an error in taking readings such as parallax error or zero error. It is advisable to check the data again.

Fig 4

Tip 5

Although I had said that it is possible to have the points two by two on both side of the line. It is not possible to have them as in fig 5 below. 

Fig 5

 

If you are in the situation that I described as in fig 5 above then you can draw the line as in the fig 6 below.

Fig 6

So if you have any difficulties drawing the line then review the five different tips above you will surely find a tip for you. Otherwise send me a picture of the points and we will see it together.

y = mx + c

We have seen in a previous post about the gradient and how it is calculated. We have also learned about the y-intercept. We are now going to combined the two concept to learn about the equation of a straight line.

All straight line can be represented by a equation that will be in the form of 

y = mx + c

We are now going to use a straight line in order to see how the equation above can be determine and how the x-coordinate or the y-coordinate could be determined if one of the two and the equation is available.

Fig 1

Fig 1 above shows a straight line graph.

The gradient of the graph can be determined using the method described here.

Two coordinates that can be used are (1,6) and  (5,18)

Thus Gradient = (18 –6)/(5-1)

                               = (12)/(4)

                                 = 3

And using the methods described here we can deduce that the y-intercept is 3.

Now the gradient = 3

and y-intercept =3

If we say that gradient = m then m= 3

and if we say that y-intercept = c then c=3

replacing the values for m and c in y= mx +c

the equation of the line becomes

y = 3x + 3

All straight lines can be represented with an equation and the way to obtain the equation is by using the method above.

We are going to use the equation to determine

(i) the value of the y-coordinate when the x-coordinate is 3

(ii) the value of the x coordinate when the y-coordinate is 15

(i) Equation of the line is y = 3x + 3 and x=3

replacing x=3 in the equation we get

y =3(3) +3

=9+3

=12 check on the graph!!!!!!

(ii) y =15

Replacing into the equation we get

15 = 3x +3

15 – 3 = 3x

12 = 3x

x =12/3

x = 4 Check on the graph!!!!!!

Pressure and upthrust

Pressure 

What is pressure?

Pressure due to a liquid

Pressure due to a liquid

We have seen in this post about the concept of pressure that a solid exerts on a surface.

Today we are going to look at the pressure that a liquid exerts on the bottom of a container in which it is present.

Fig 1 below shows a container containing an unknown orange liquid. The depth of liquid in the container is h. The density of the liquid is denoted by the letter ρ.

Fig 1

Fig 2 below represent the same container but this time in 3 dimensions. The container is in the form of a cuboid. The liquid will thus be in the form of a cuboid with cross-sectional area and height h.

Fig 2

Now how do you obtain the pressure that the liquid is exerting on the bottom of the container?

The equation for pressure is

Pressure = Force Applied /Surface area

              = Force applied by liquid/surface area over which pressure is applied

              = Weight of liquid /Cross-sectional area A

              = mg /A

               = (Volume of liquid *density*g)/A

                = (Ah *ρ)*g/A

                 = h*ρ*g

                 = hρg

Where g is the acceleration due to gravity.

Example

A bottle of water of density 1000 kg/m³ has water of depth 0.10 m in it. If the acceleration due to gravity,g, is 9.81 m/s² , calculate the pressure due to the water on the bottom of the container.

Pressure due to water =hρg

= 0.10*9.81*1000

=981 Pascal (Pa)

Practical skills

The gradient of a line.

The y-intercept of a line. 

y=mx+c

The line of best fit

What is the y-intercept?

We have seen in a previous post the gradient of a straight line graph. Today we are going so have a look at the y-intercept.

Now as you know a graph is composed of two axes. The y-axis and the x-axis as shown in fig 1.

Fig 1

Now in order to find the y-intercept you will need a straight line that passes through the y-axis, i.e it intercept the y-axis,  as seen in fig 2 below.

Fig 2

As you can see in fig 2 above all three lines crosses the y-axis. As a result the three lines would have a y-intercept.

How to obtain the y-intercept of a straight line?

1. If the x-axis starts at 0

As you can see in fig 3 below the point at which the line crosses the y-axis is at the y=2 coordinate. Hence the y-intercept is 2.

We can thus define the y-intercept as being the value of the y-coordinate when the x-coordinate is 0. 

Fig 3

2. If the x-axis does not start at 0

As you can see in fig 4 below the x-axis does not start at 0. Hence as you may have guess wrongly the y-intercept is not 2 since according to the definition the y-intercept is the y-coordinate when the x-coordinate is 0.

So how do you obtain the y coordinate.

Fig 4

You will first have to calculate the gradient of the line using the method described in this post.

The gradient in this case is 1.

You will use the equation y = mx + c  and a coordinate on the line in this case (3,2).

y = 2

x=2

gradient = m =1

Hence the only variable left is c, the y-intercept.

3 = 1*2 + c

c = 3 – 2 =1

The y-intercept of the line is thus 1.

You should thus be very careful to check that the x-axis starts with 0 or does not start with 0 so as to choose which of the two methods to use.